Euler's Formula
Most importantly for us, the formula for e ix leads directly to Euler's formula. π is the half-circle arc:
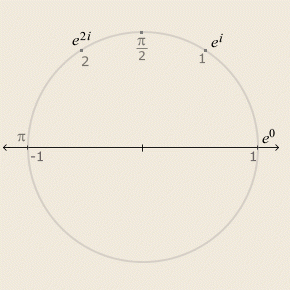
So at last:
e iπ = cos (π) + i sin (π)
e iπ = −1 + i×0
e iπ = −1
e iπ = −1 + i×0
e iπ = −1
Q. E. D.
We're not through mining ex yet. A little calculation with 2i, 3i and so forth in the formula for ex will show that:
And in general:
This shows, at last, that ex indeed combines the virtues of the sine and cosine functions into one: the perfect tool for compact representation of complex numbers. A complex number a + bi is some point that marks off an arc length of θ on a circle, and can be written:
which is the same as:
Put another way, the imaginary powers of e trace the unit circle (see the diagram at right).
Most importantly for us, the formula for e ix leads directly to Euler's formula. π is the half-circle arc:

So at last:
Q. E. D.